Math with Ms. Routt- Computational Fluency

The third component of my math block is computational fluency. According to Robert Ashlock (2010), author of Error Patterns in Computation: Using Error Patterns to Help Each Student Learn, today’s mathematicians “need to be able to use efficient and accurate methods for computing.” To meet this goal, I include this five minute segment to encourage fact fluency and flexibility with composing and decomposing numbers.

Over the years, I have done this many different ways depending on the grade level and ability level of my students. Here are a few ways I have structured this time over the years:

1. Counting and Grouping Methods: With younger students, I begin the year using Greg Tang’s math picture books to support the development of this skill.

“Snail Parade” from The Grapes of
Math written by Greg Tang and
illustrated by Harry Briggs.
Picture from:
http://eduscapes.com/nonfiction/10.htm

The “Snail Parade” picture above is from Greg Tang’s book titled, The Grapes of Math. I put this page under the document camera for about 5 seconds. Students are then expected to determine how many snails are on the page. In the beginning, students attempt to count them one by one, but they quickly realize there is not enough time to count them all. They then move to other grouping techniques, such as “I see two rows of five and three rows of four, or 10 + 12, so there are 22 snails.” Another student may say, “I see five rows of five with three snails missing, or 25 – 3, so 22 snails.” In both cases, these methods provide a very rich opportunity for me to extend the students’ thinking to numerical equations, such as (2 x 5) + (3 x 4) = 22 or (5 x 5) – 3 = 22. This provides a good foundation for the work with order of operations that will come later.

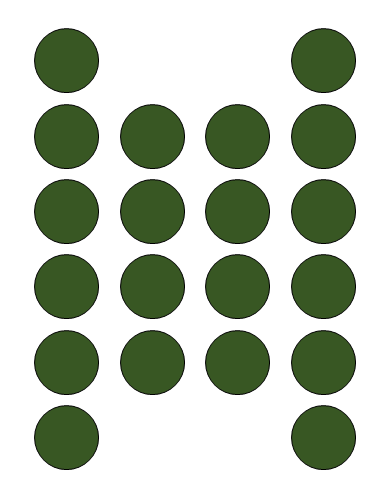
At some point, I switch to making dot pictures with transparent chips or dot stickers. This allows me to create larger array-like pictures that are easier for the students to decompose quickly. For example, in the picture to the left, students may notice four rows of four plus the four corners, six rows of four minus four, or two rows of six plus two rows of four. In all of the cases, the students have identified 20 dots. After hearing the verbal descriptions, I connect them to numerical expressions.

Over time, I noticed my students’ fluidity with numbers and their ability to compose and decompose numbers quickly improves.

2. Fact Fluency: With middle grades students, the primary focus for fact fluency is learning multiplication and division facts. To help the students meet this goal, I use mini-lessons and frequent practice to increase fact fluency. Check out my Engaging Mathematics: Learning Multiplication Facts here.

3. Using Mental Math: With my upper grades students, I use this time to practice using mental math to solve simpler multiplication and division problems. For example, for 52 x 7, it can be decomposed into 50 x 7 and 2 x 7 for a final product of 364. Practicing mental math computations increases computation speed and supports fluidity with numbers.