Math Talk Routines that Work!

As I recorded the problem on the board, I said, “Find the product for 24 x 8 using mental math.”

After a few moments, Raymond raised his hand to signal he was finished. 

I walked over to his desk. 

Raymond slyly slid over his board so I could see his work. 

I then said, “Thank you, but this is not what I asked you to do.”

He glared at me with a puzzled look and a know-it-all smile.

“Try again,” I said quietly. 

Finally, after several failed attempts, he looked at me and said, “There is no other way.”

At that moment, David walked over to Raymond and said, “This is what she means.” 

He proceeded to show his new classmate how to break the 24 into 20 and 4 and then multiply each part by 8 to get 160 + 32 = 192.

I couldn’t believe my eyes! 

David, one of my lowest students, was teaching my highest achieving student how to multiply using a mental math strategy. 

I was beaming– talk about a proud teacher moment!

How It All Began

Before number talks were a thing, I regularly posted problems for my students to solve using mental math strategies. It was fun to hear the students sharing their strategies and competing with each other to think of a strategy no other student shared. 

In fact, I started this routine with pictures from Greg Tang’s math picture books so students built a habit of looking at the images and finding ways to divide the quantities so they were easy to count. 

This math talk routine became a staple in my classroom and one I could not only use to get my students talking mathematically, but one that could help me reinforce numerical reasoning skills at the same time. 

What are Math Talk Routines?

A math talk routine is a daily or weekly activity used to engage students in mathematical communication.

Over the years, I’ve heard some teachers say they feel like they have to have a grand plan for using math talk in the classroom. While it is important to understand your purpose and what you hope to accomplish with your math talk session, there are some simple routines you can put into place that will provide you with a structure. All you have to do is supply the content and the tools students need to communicate mathematically. 

Not sure how to get started? I’ve got you covered! 

Here are five simple routines you can put into place that will provide you with a structure for using math talk in the classroom. 

Math Talk Routines

A. Who’s Correct?

Who’s Correct is a strategy that requires students to analyze and make sense of the thinking of others. What I love about this strategy is that students do not just solve the problem, they must select a correct, or incorrect, response and provide a justification for the thinking of another. 

Here’s How It Works:

1. Present a problem situation to the class along with two to four student responses and supporting reasoning. Note: This task works best with student responses that reveal a common misconception or misunderstanding.

2. Give students time to think, determine the person with correct thinking, and provide a justification.

3. Designate one corner of the room for each response. Have students go to the corner that represents the response they selected.

4. Give each corner an opportunity to discuss why they believe their student’s response is correct.

5. Have each corner share their reasoning with the class. 

B. Fact or Fib

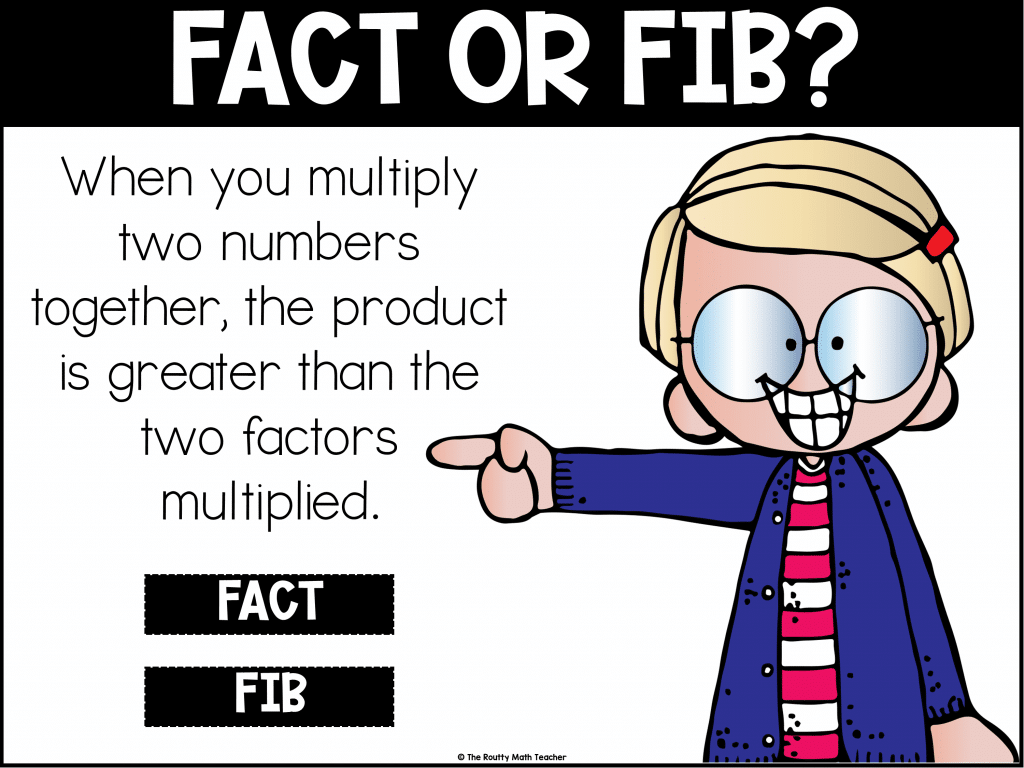
Fact or Fib has been used in a variety of ways for several years. It’s a spin-off of the oh-so-familiar true or false task but adds a new context. To make the most of this task, teachers encourage students to be fact checkers as the learning here comes from the justification piece. It is not enough for students to respond with simply “fact” or “fib”. They must know why the statement is a fact or a fib and be able to communicate their thinking in pictures, words, or numbers. 

Here’s How It Works:

1. Group students in groups of 3 or 4.

2. Give each student a “Fact or Fib” desk tent or have students write “fact” and “fib” on individual index cards.

3. Give the students a statement based on the current standard or skill. For example, “When you multiply two numbers together, the product is greater than the two factors multiplied.”

4. Give each student time to determine whether the statement is a fact or a fib. Then have students create examples or counterexamples to justify their response on a whiteboard.

5. Once all students have written justifications, each student displays his/her desk tent to show whether they believe the statement to be a fact or a fib.

6. In turn, each student states whether the statement is a fact or a fib and gives his/her justification.

7. After all group members have shared, the group should come to a consensus and have several examples or counterexamples to support the group’s decision.

8. Each group shares their consensus with the class.

9. After all groups have shared, verify and/or clarify student responses addressing misconceptions where needed.

10. Repeat the process with another statement.

C. Sometimes, Always, or Never

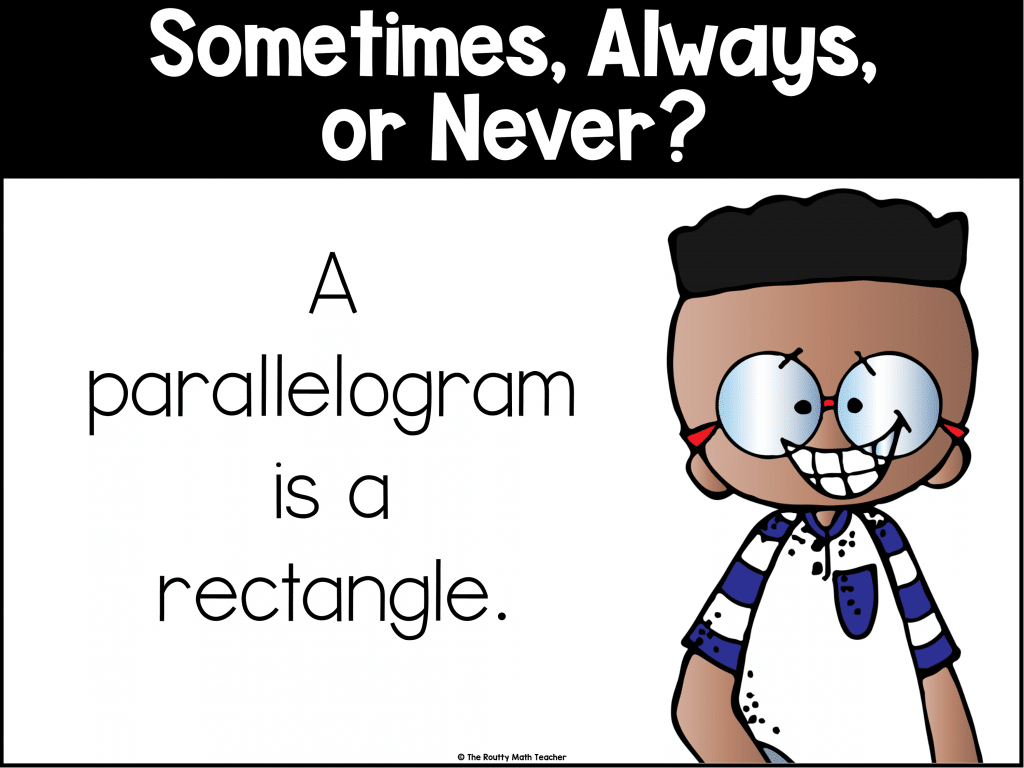
I first learned about Sometimes, Always, or Never a few years back. As a lover of reasoning, I fell in love with this strategy from the first day I learned about it and I have been using it and adapting it ever since. What I love most about this strategy is the way it makes students think. To determine the correct response, students must consider all of their options. They must decide if the statement is sometimes true, always true, or never true. And, to do this, students must be able to select instances of and then try to create a generalization for the concept.

Here’s How It Works:

1. Group students in groups of 3 or 4.

2. Give the students a statement based on the current standard or skill. For example, “A parallelogram is a rectangle.”

3. Give each student time to determine whether the statement is “always true,” “sometimes true,” or “never true.” Then, have students create examples or counterexamples to justify their response on a whiteboard.

4. Once all students have written justifications, each student states whether the statement is “always true,” “sometimes true,” or “never true” and gives his/her justification. 

5. After all group members have shared, the group should come to a consensus and have several examples or counterexamples to support the group’s decision.

6. Each group then shares their consensus with the class.

7. After all groups have shared, verify and/or clarify student responses addressing misconceptions where needed.

8. Repeat the process with another statement.

D. Estimation 180

Estimation 180 is a website which includes a plethora of images from the real world where students have to estimate some quantity in regards to the amount represented in the picture. This bank of content is so large, there is a challenge for each day of the school year, including themed challenges for various holidays, such as Halloween and Valentine’s Day. 

Here’s How It Works:

1. Choose an image to present to the class. 

2. Ask students to use the clues to develop a strategy to estimate the quantity displayed. 

3. Have students use their understanding of the task to create a range of possibilities, identifying what’s too low or too high. 

4. Reveal the real quantity and have students compare their estimates to the actual quantity,  refining their thinking as needed. 

Note: Be sure to download the Estimation 180 handout to help your students organize their thinking. 

E. Which One Doesn’t Belong

The idea behind Which One Doesn’t Belong is simple but so brilliant. Students are presented with a set of pictures and asked to decide which one doesn’t belong in the set. The beauty of this challenge– there are multiple ways to look at the images and ways to decide how to exclude an item from the set. There is no right or wrong answer! As long as students can justify their response, their answer is correct. 

Here’s How It Works:

1. Present a picture or image with multiple components. 

2. Have students discuss which picture or element of the picture does not belong. 

3. Ask students to share their reasoning and compare it to the reasoning of other students. 

Getting Started

The best way to get started promoting math talk in the classroom is to choose an activity and use it! I recommend embedding a few opportunities each week for students to stretch their brains and participate in math talk. Try introducing one routine at a time until students are comfortable before adding additional routines. 

Additionally, while you may choose to use a routine in isolation, using a routine to deepen and extend student understanding of the content you teach is a powerful way to enhance student learning. 

Want to know more about math talk moves and how to use them to enhance math talk? 

Check out this blog post and download my Math Talk Moves poster using the form below.

Sound Off!

Have you tried any of these strategies? If so, share your experience in the comments section below.